FLUCTUATING LOAD DESIGN:INTRODUCTION

STUDY OF FLUCTUATING STRESSES :WHY IT IS IMPORTANT IN DESIGN???

Fluctuating stresses??? WHAT, HOW AND WHY????

Till now, IN THE PREVIOUS BLOGS, we learned about static loading and its related stresses.

IN CASE IF YOU MISSED IT , PLZ REFER TO THE LINK BELOW:

https://mechomotive.com/wp-admin/post.php?post=3355&action=edit

Static loading is that loading which does not change w.r.t magnitude and direction.

But we see that many practical applications seemed to work on specific loading conditions. These acting forces changed in magnitude OR direction OR both.

These forces , so called fluctuating forces depend on magnitude OR direction OR both magnitude and direction.

FLUCTUATING STRESS: The stresses produced from fluctuating forces and loading system.

NEED OF FLUCTUATING STRESS STUDY:

Nowadays, many of the industries work on fluctuating stress and loads.

It came into study by the following observation:

“About 80% of the components failed due to fatigue failure occurring due to fluctuating stresses.”

So, study of fluctuating stresses came needed to make design safer.

Since, cyclic stress variations were irregular and non-analytic, various models came in suggestion for the study.

AT LAST, Sine curve came to be taken as the easiest and popular model.

This model was based on Stress -Time relationship.

CYCLIC STRESS MODELS – BASED ON “SINE CURVE MODEL”

Before going for cyclic stress study , Let’s have a glance on some important terms

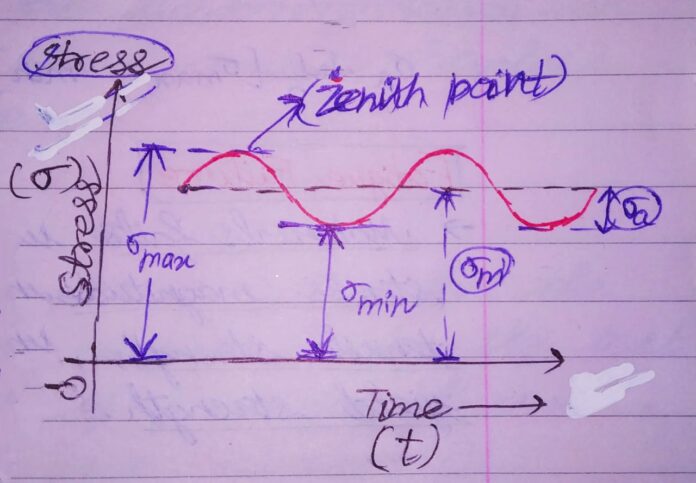
1. MAXIMUM STRESS: Maximum stress of the system. Denoted by σ_max .

2. MINIMUM STRESS: Minimum stress of the system. Denoted by σ_min.

3. MEAN STRESS: Sum average of the MAX. and MIN. stress of the system. Denoted by σ_m.

σ_m= σ_max + σ_min/2

4. VARIABLE STRESS/STRESS AMPLITUDE: Average of the difference of the MAX. and MIN. stress of the system. Denoted by σ_v.

σ_v= σ_max – σ_min/2

There are main 3 important mathematical models for Cyclic Stresses :

1. FLUCTUATING STRESSES/ ALTERNATING STRESSES :

These stresses show changes in a sinusoidal manner w.r.t time. It has some maximum value as well as minimum value stress.

So, there is a fixed mean stress value as well as variable stress value. These stresses can be – Tensile, Compressive or Partial tensile and partial compressive.

Here, σ_max & σ_min ≠0

Therefore , σ_m & σ_v ≠0

2. REPEATED STRESSES:

These stresses show changes in a sinusoidal manner w.r.t time. But, here the variation starts from the origin (i.e 0,0).

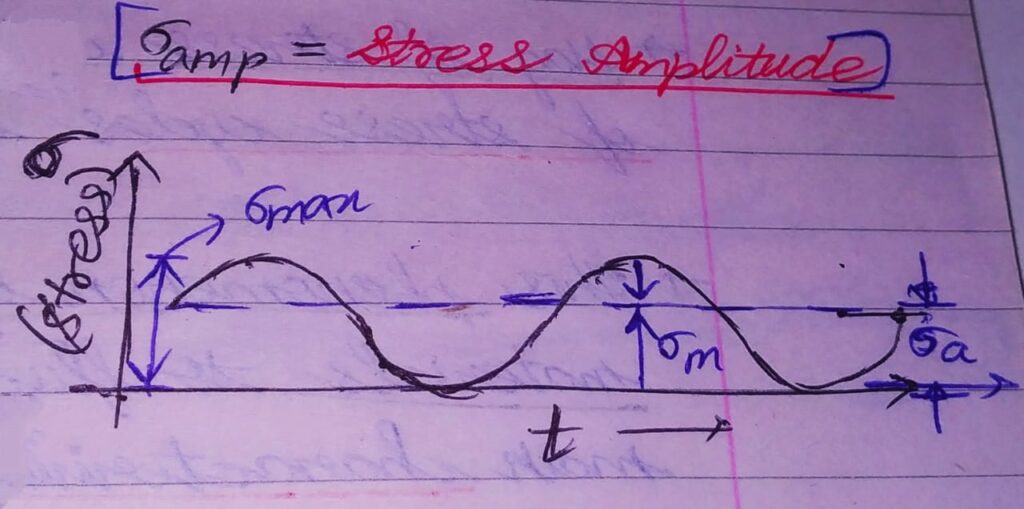
Therefore, σ_min=0 & σ_max= Definite value

σ_m= σ_max + σ_min/2 = σ_max/2

σ_v= σ_max – σ_min/2 = σ_max/2

3. REVERSED STRESSES :

These stresses show changes in a sinusoidal manner w.r.t time. But , here the maximum value and minimum value stresses are equal in magnitude ,but opposite in direction.

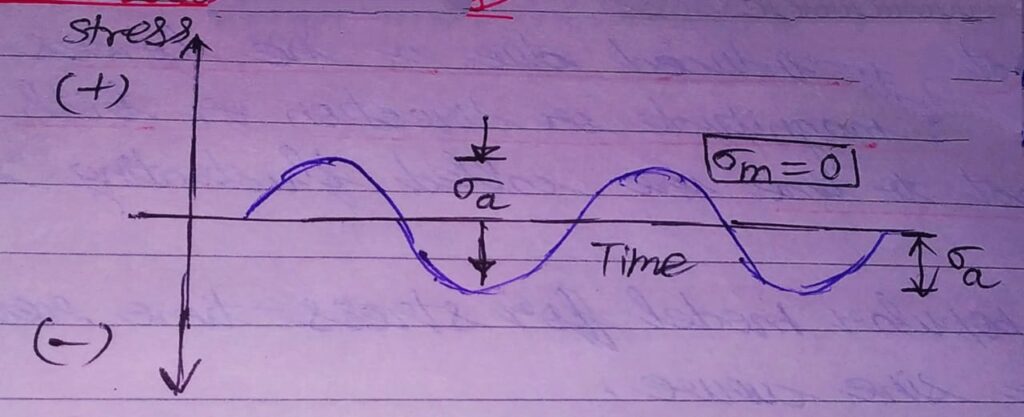
Therefore, σ_min=-σ & σ_max=+σ

σ_m= σ_max + σ_min/2 = 0

σ_v= σ_max – σ_min/2 = σ

In fluctuating stress analysis , tensile stress is taken as positive, while compressive stress is taken as negative.