THEORIES OF FAILURE 3 – STRAIN ENERGY

NEED OF ENERGY STUDY IN TOF

Study through concept of stress and strain made the Analysis of Theories of failure problematic and inaccurate.

So , the engineers now decided to go through the *Energy ideology* and its concept.

So , STRAIN ENERGY is the best energy analogy related to COMPLEX STRESS SYSTEM induced bodies.

STRAIN ENERGY

Energy induced or work done by the load in a loaded body.

Here , energy gains or losses due to heat are considered negligible.

Denoted by U.

U = P δ/2 U/volume = σε/2 P = Load δ= elongation

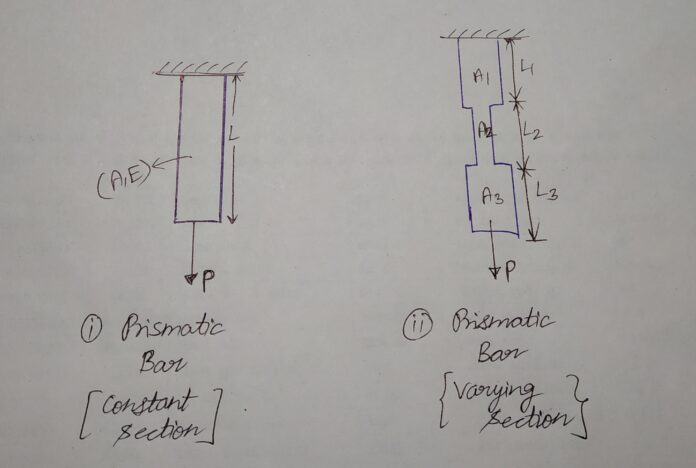
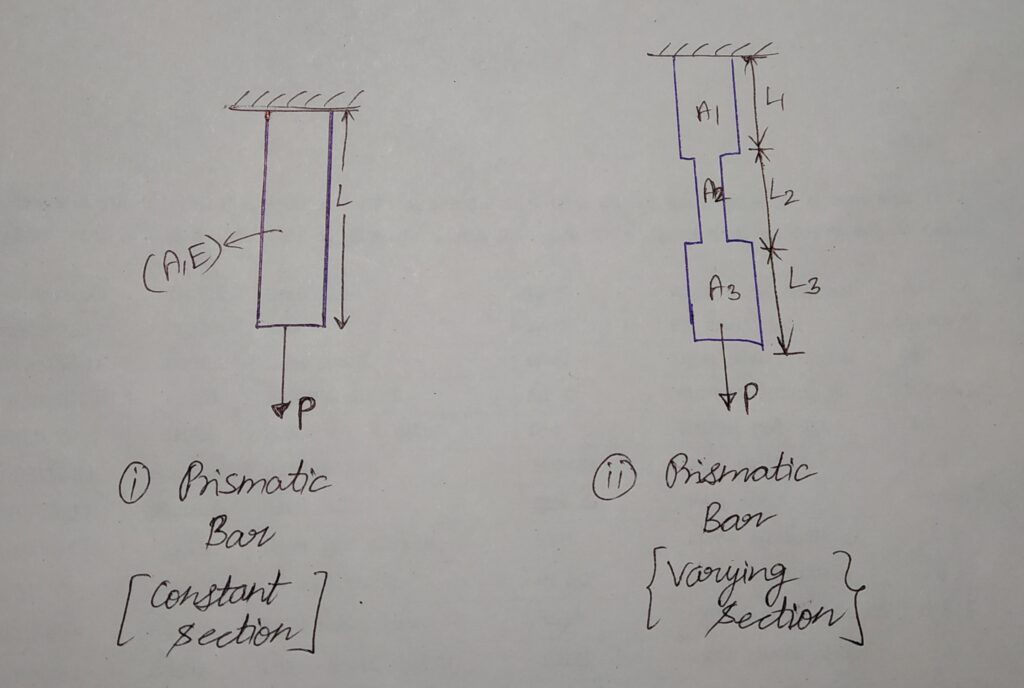
a) For axially loaded prismatic bar (Constant cross section)

At δ=PL/AE U = P²L/2AE

b) For axially loaded prismatic bar (varying sections)

U=P²/2E { (L1/A1) + (L2/A2) + (L3/A3)+……………….}

c) Non prismatic bar(varying cross section) with varying axial load

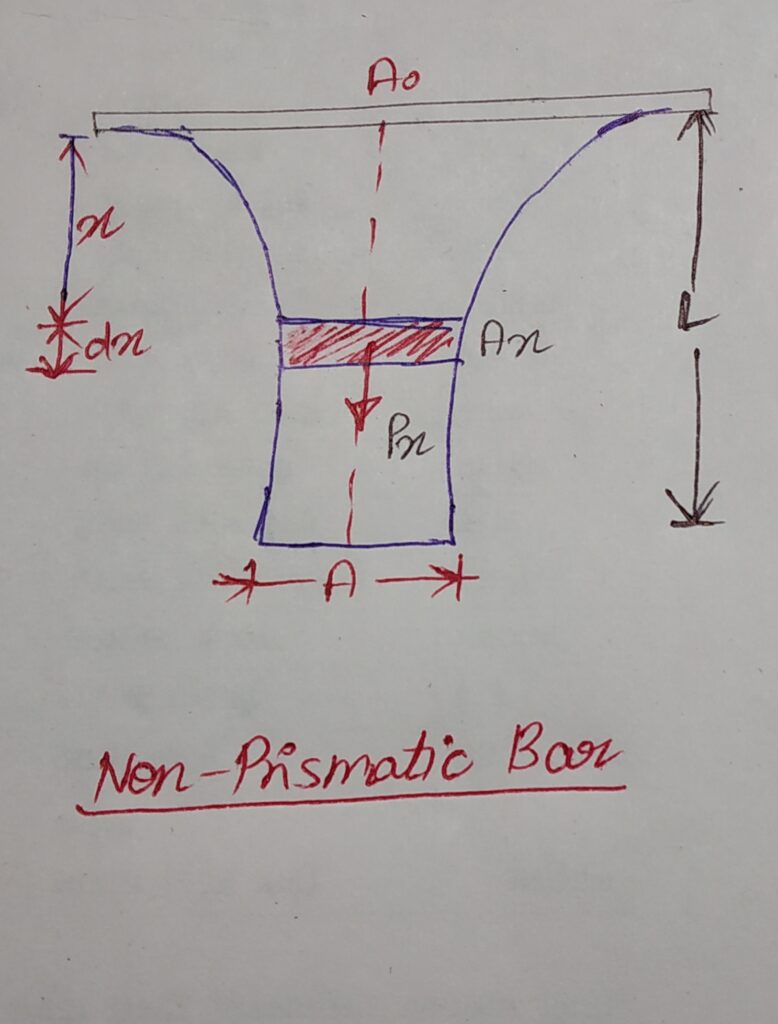
U= ∫ P_X² .dx /2EA_X Integrated along its length (0 to L)

THEORIES OF FAILURE 2 : COMPLEX STRESS

REFER TO THE ABOVE LINK FOR FIRST 3 THEORIES OF FAILURES

*THEORIES OF FAILURE ( CONT.)

4.MAXIMUM STRAIN ENERGY THEORY / (MSET) (HAIGH AND BELTRAMI THEORY):

* HAIGH AND BELTRAMI developed this theory.

According to this theory ;

Under complex stresses, the material fails when the max. strain energy/volume crosses the limit of strain energy/volume at yield point at uni-axial loading.

U > σ_y² /2E

For safe design : U ≤ (σ_y/FOS)² /2E

# Always FOS=1 ,whenever value of FOS not given in the question.

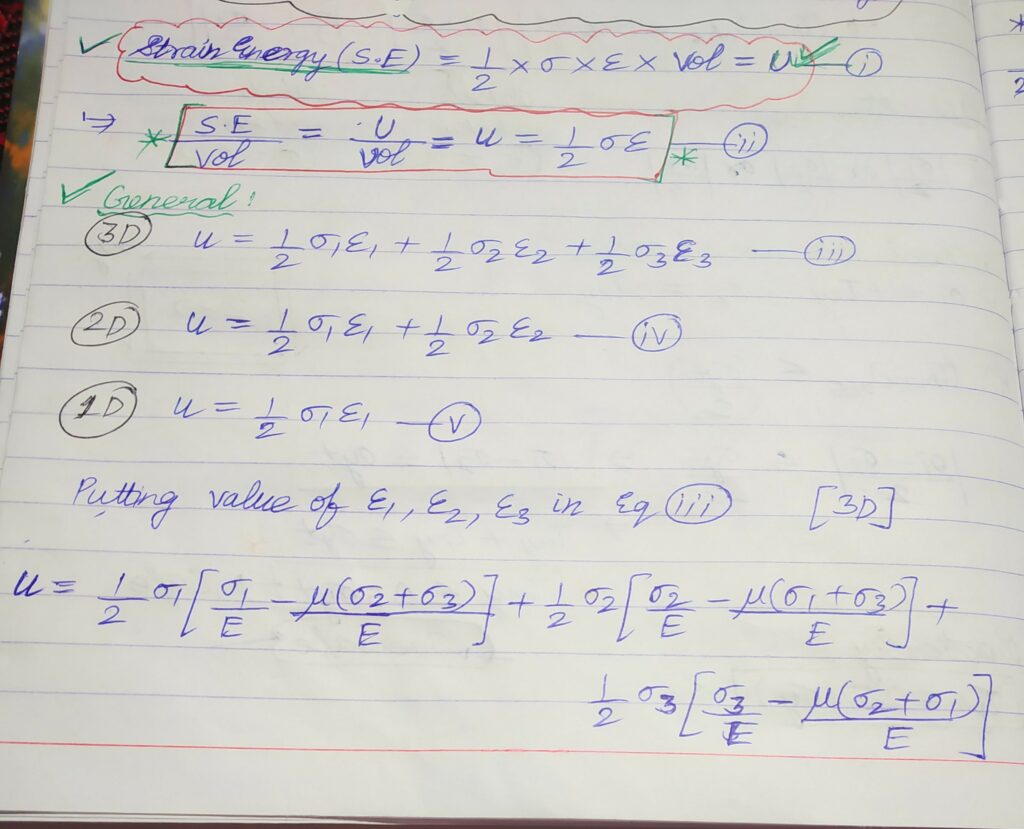
LIMITATIONS :

1.Although inaccurate w.r.t practical conditions, but accurate than of other theories discussed.

2.Cannot be applied to brittle materials. Because it has varying elastic limit stress values at tension and compression.

3.Can be only applied for ductile materials as limit values are constant.

5. MAXIMUM SHEAR STRAIN ENERGY THEORY/(MS_NET)/DISTORTION ENERGY THEORY/VON MISES – HENKY THEORY

* VON MISES AND HENKY developed this theory.

According to this theory;

Under complex stresses, the material fails when the induced max. shear stain energy/volume crosses the shear strain energy/ volume at yielding under uni-axial loading.”

Shear strain energy (Us)/ volume = or > (shear strain energy(Us)/volume)_{yield point(1D)}

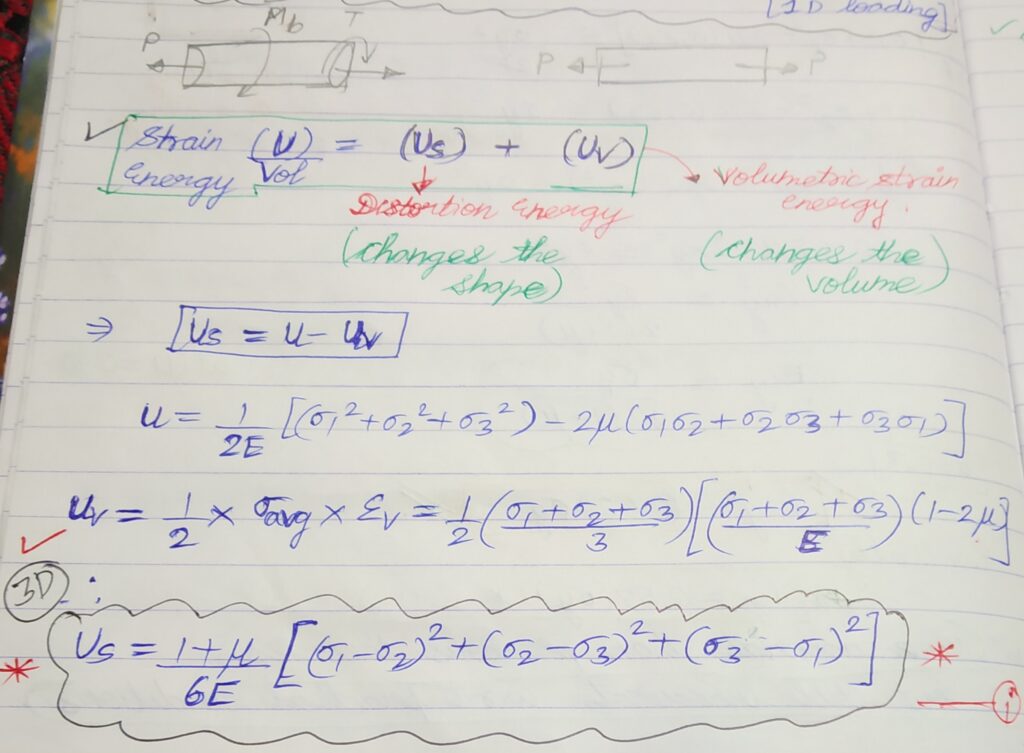
The most accurate resullt has been found.

Huraayyyyyy!!!!!!!!!!!!!!

PROS :

1.Best theory for ductile materials study and design.

2.Gives accurate result in pure shear condition same as experimental result.

LIMITATIONS :

1.Cannot be applied for brittle materials study due to varying limit zones.

2.Not applicable on hydro-static pressure system(No shear system).

FOLLOWING THEORIES OF FAILURES ARE BEEN DISCUSSED COMPLETELY.

https://mechomotive.com/wp-content/uploads/2021/02/TOF-NUMERICAL.pdf